

In our next segment, we will introduce the box method for handling several of the integrals left unsolved in this post. This says, for example, that when confronted with, differentiate the and integrate. Some people enjoy a good mnemonic to memorize the order. This list represents a good order in which to choose your u in the following sense: if you have two functions, whichever comes first in the above list should be your u. Logarithms Inverse-Trig Algebraic Trig Exponential If we also include trigonometric functions and exponential functions, the rule of thumb is: Otherwise, you should differentiate the polynomial. So when does it pay to put the polynomial on the right? Whenever the derivative of the other function changes it into an algebraic function, it will be right to integrate the polynomial.

On the other hand, if we put the on the left, the derivative will return and the natural logarithm is gone.
#Tabular integration by parts how to
Although we did integrate in our first post, it gave an answer of and we don’t want to integrate that since it we don’t know how to integrate (in a future post, we will resolve this last problem directly).

In fact we have done more, but we’ll have to wait until the next post to resolve this.Īnother example is. However, if you put the on the left, we get:Īnd at the very least we have gotten rid of the. You would now get:Īnd be forced to integrate the monstrosity on the right. Let’s say you just happen to know the antiderivative of is (I didn’t, although I can use integration by parts to figure it out!). If we split this up using our ‘rule’ to always put the polynomial on the left, then we are forced to integrate. We implicitly saw this in the first post, but let me give you a couple of more explicit examples. It turns out that there are several examples where this is precisely the wrong thing to do. This suggests that we should always put a polynomials on the left so that it doesn’t go up in degree. Ah, but what if we start with the cosine on the left and then switch it up? Oh, yeah, we’ll just get back what we started with.
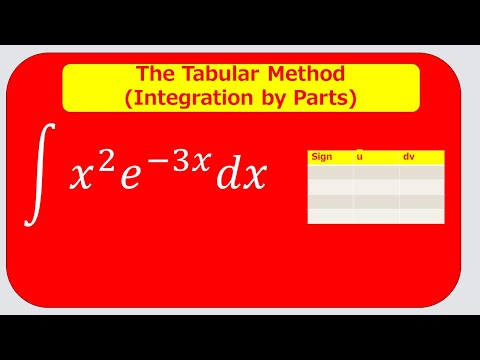
Since the product of the terms in the last line of the table is what we will need to integrate, doing it this way just makes things worse. Let’s take a look at what happens if we switch it up.Įgad, Dave is right. ” Then when you work it through, everything cancels out and we’re back to the original problem. What if we switch up our u and dv this time? Let’s let and. I usually make a show of how sometimes the order does matter…That is, I’ll let and the first time and then go through it and say something like, “Well, that didn’t get us much of anywhere.
#Tabular integration by parts series
Über-reader CalcDave wrote in the comments to the last post in this series that, In this post, we will consider the following question: As integration by parts requires the making of a choice-which is your u and which is your dv-how can we make this choice so that the resulting integral is easier to compute? We introduced integration by parts as a way to compute antiderivatives of a product of functions and we saw how certain integration by parts problems are handled more efficiently with the so-called tabular method (or, in Stand and Deliver, the “tic-tac-toe” method). In the first two installments of this series


 0 kommentar(er)
0 kommentar(er)
